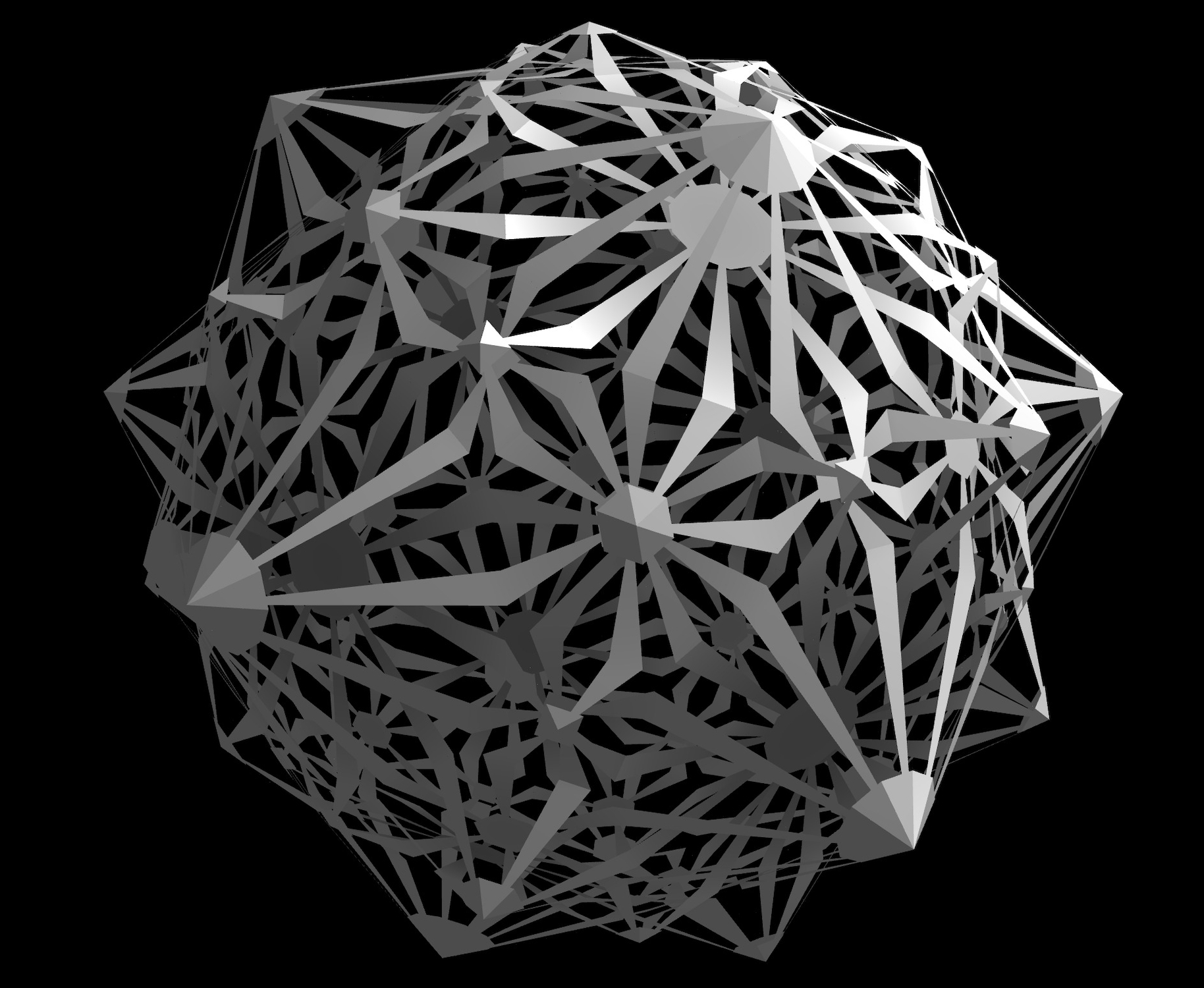
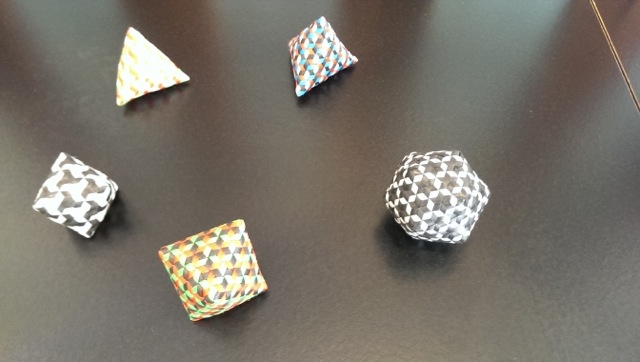
Mathematics and physics are powerful tools for understanding and describing the world around us. From the motion of celestial bodies to the behavior of particles at the quantum level, these disciplines help us unravel the mysteries of the universe. One fascinating area of study is the mathematical modeling and simulation of galaxy polyhedra.
Galaxy polyhedra are geometric structures that can be used to approximate the shape and structure of galaxies. They provide a simplified representation of the intricate patterns and formations found in these cosmic objects. By employing mathematical models and simulations, scientists can gain insights into the formation and evolution of galaxies, as well as their properties and behavior.
Mathematical models of galaxy polyhedra involve the use of concepts such as graph theory, topology, and geometry. These models allow researchers to study various aspects of galaxies, such as their clustering, connectivity, and spatial distribution. By analyzing the properties of galaxy polyhedra, scientists can investigate the patterns and dynamics of galaxy formations, as well as the impact of different factors on their structure.
Simulations play a crucial role in understanding the behavior of galaxy polyhedra. By creating virtual environments that mimic the conditions of the universe, scientists can observe and analyze the interactions between galaxies and the forces that shape their structures. Simulations enable researchers to test different hypotheses, make predictions, and refine their understanding of the complex processes occurring in the universe.
The Galaxy Polyhedra are mathematical models that represent the structure of galaxies. They are composed of interconnected polygons and provide a visual representation of the complex nature of galaxies.
Galaxies are vast systems of stars, gas, and dust that are held together by gravitational forces. They come in various shapes and sizes, with spiral, elliptical, and irregular being the most common types. Understanding the structure of galaxies is essential for astronomers to study their formation, evolution, and other properties.
The Galaxy Polyhedra offer a unique way to visualize and study the intricate patterns and connections within galaxies. By representing galaxies as interconnected polygons, astronomers can gain insights into their geometry, symmetry, and connectivity. This helps in identifying clusters, groups, and other arrangements of stars within a galaxy.
Furthermore, the Galaxy Polyhedra can be used to simulate various scenarios and phenomena within galaxies. By manipulating the model's parameters, astronomers can explore the effects of interactions between stars, as well as the evolution of gas and dust within galaxies. This allows for a better understanding of the processes that shape galaxies over time.
One of the key aspects of using models like the Galaxy Polyhedra is their ability to provide a visual representation that makes complex concepts more accessible. They allow astronomers to communicate their findings to a wider audience and spark interest and curiosity in the general public.
Galaxy polyhedra have always fascinated scientists and mathematicians with their intricate shapes and complex structure. These celestial objects, also known as cosmic polyhedra, are three-dimensional structures that resemble the polyhedra we encounter in geometry. However, galaxy polyhedra are not man-made constructions; they are natural formations that occur in the vast expanse of the universe.
Studying the mathematical basis of galaxy polyhedra requires a deep understanding of geometry, topology, and mathematical modeling techniques. Mathematicians and scientists have developed various mathematical models and simulations to explore and understand the underlying principles that govern the formation of these cosmic objects.
Geometry plays a crucial role in understanding the shapes and structures of galaxy polyhedra. The study of the geometrical properties of these celestial objects helps us classify them and identify their unique characteristics. By examining the angles, lengths, and symmetries of their faces and edges, mathematicians can create mathematical models that accurately represent these cosmic entities.
Topology, on the other hand, focuses on the properties of space that are preserved under continuous transformations. By studying the topology of galaxy polyhedra, mathematicians can understand the connectivity and relationships between different parts of these cosmic structures. This knowledge aids in the creation of simulations that can mimic the formation and evolution of galaxy polyhedra.
Mathematical modeling involves the creation of mathematical equations and algorithms that describe the behavior and properties of galaxy polyhedra. These models can take various forms, including differential equations, network models, and computational simulations.
One commonly used approach is differential geometry, which uses differential equations to describe the curvature and deformation of space. By applying these techniques to galaxy polyhedra, mathematicians can create sophisticated models that capture the intricate shapes and dynamic properties of these cosmic objects.
Another approach involves using network models, which represent galaxy polyhedra as interconnected nodes and edges. By analyzing the connectivity and interactions between these elements, scientists can gain insights into the underlying structural patterns and properties of galaxy polyhedra.
Computational simulations, on the other hand, involve the use of computer algorithms to recreate and study the formation and evolution of galaxy polyhedra. Through these simulations, mathematicians can investigate the impact of various factors, such as gravitational forces and interstellar gas dynamics, on the shape and structure of galaxy polyhedra.
By exploring the mathematical basis of galaxy polyhedra through various modeling and simulation techniques, scientists and mathematicians can deepen their understanding of these celestial objects and gain valuable insights into the complex nature of the universe.
In recent years, the exploration of mathematical models and simulations has become increasingly important in the study of galactic structures. One fascinating area of research is the simulation of galaxy polyhedra in virtual environments.
Galaxies are vast collections of stars, gas, and dust held together by gravity. They come in different shapes and sizes, ranging from spiral galaxies like our Milky Way to elliptical and irregular galaxies. Understanding the dynamics and evolution of galaxies helps astronomers gain insights into the formation and evolution of the universe.
Simulating galaxy polyhedra involves creating three-dimensional models that represent the overall shape and structure of galaxies. These models can be used to visualize and study various aspects of galaxy formation and evolution.
One approach to simulating galaxy polyhedra is by using advanced computer modeling techniques. Scientists can input data about the composition, distribution, and motion of stars, gas, and dust in a galaxy, and then use algorithms to generate a realistic virtual model. By adjusting the parameters of the simulation, researchers can observe how different factors affect the overall shape and structure of the galaxy.
Virtual reality (VR) technology has also been utilized to simulate galaxy polyhedra. By immersing users in a virtual environment, VR allows for a more interactive and immersive exploration of galaxy structures. Users can navigate through simulated galaxies, observe their intricate details, and gain a deeper understanding of their formation and dynamics.
The simulations of galaxy polyhedra have numerous applications in astrophysics. They can help researchers study galaxy mergers, understand the formation of galactic arms, investigate the effects of supermassive black holes on galaxy structures, and explore the connections between galaxies and their surrounding environments.
In conclusion, simulating galaxy polyhedra in virtual environments is an exciting and promising area of research. The combination of mathematical models and computer simulations allows scientists to explore the complexities of galactic structures and gain deeper insights into the nature of our universe.
Mathematical models and simulations play a crucial role in understanding the vast complexity of the universe. They provide scientists with a powerful tool for studying and predicting the behavior of various phenomena, from the smallest particles to the largest galaxies.
One intriguing application of mathematical models and simulations is in the study of the structure of galaxies. By applying mathematical equations to various observations and data, scientists can create simulations that mimic the gravitational interactions within a galaxy. These simulations help researchers understand how galaxies form, evolve, and interact with one another. They also provide insights into the distribution of dark matter and the formation of spiral arms and other features.
Mathematical models and simulations are also used to predict the future evolution of the universe. By combining the laws of physics and astronomical observations, scientists can create simulations that simulate the expansion of the universe, the formation of cosmic structures, and the behavior of dark energy, among other things. These simulations allow astronomers to test different hypotheses and scenarios, helping them refine our understanding of the past, present, and future of the universe.
1. Enhances our understanding of complex systems
2. Provides a virtual laboratory for testing hypotheses
3. Allows for the exploration of extreme conditions that are difficult to observe directly
4. Helps scientists make predictions and design future experiments
In conclusion, mathematical models and simulations are invaluable tools for understanding the universe. They enable scientists to explore complex phenomena, predict the future evolution of the universe, and refine our understanding of fundamental concepts. By combining mathematical rigor with observational data, these models help us unlock the mysteries of the cosmos and deepen our knowledge of the world around us.
In recent years, the study of galaxy polyhedra has gained significant attention in the field of mathematics. These intricate structures, formed by connecting vertices with edges and faces, can exhibit complex patterns and symmetries that have fascinated scientists and mathematicians alike. To better understand these structures and their properties, computer programs play a crucial role in modeling and simulating galaxy polyhedra.
Computer programs provide a powerful tool for creating and visualizing mathematical models of galaxy polyhedra. By inputting specific parameters such as the number of vertices, edges, and faces, these programs can generate three-dimensional representations of these structures. This allows researchers to explore various properties of galaxy polyhedra, such as their symmetry groups, surface area, and volume.
Furthermore, computer programs enable the creation of interactive models that can be manipulated in real-time. Users can rotate, scale, or morph the shape of a galaxy polyhedron, providing a dynamic visual experience. This interactivity allows researchers to gain a deeper understanding of the geometry and topology of these structures, leading to new insights and discoveries.
In addition to modeling, computer programs also enable the simulation of galaxy polyhedra. These simulations involve applying various mathematical algorithms to study the behavior and dynamics of these structures. For example, programs can simulate the growth or transformation of a galaxy polyhedron over time, allowing researchers to investigate how these structures evolve and change under different conditions.
Simulations can also be used to analyze the structural stability of galaxy polyhedra. By subjecting these structures to external forces or perturbations, researchers can study their resistance to deformation and determine their mechanical properties. This information is crucial for understanding the physical properties of real-world objects that exhibit similar structural features.
One application of simulated galaxy polyhedra is in materials science, where researchers study the mechanical properties of materials with complex microstructures.
Another application is in computer graphics, where simulated galaxy polyhedra can be used to generate visually appealing 3D graphics and animations.
Furthermore, simulated galaxy polyhedra have applications in the field of architecture, where they can inspire the design of innovative structures with unique geometries.
In conclusion, computer programs play a crucial role in modeling and simulating galaxy polyhedra. They enable the creation of mathematical models and interactive visualizations, allowing researchers to explore the properties and symmetries of these intricate structures. Simulations powered by computer programs provide insights into their dynamics and mechanical behavior, leading to applications in various fields such as materials science, computer graphics, and architecture.
Mathematical models and simulations have revolutionized our understanding of complex phenomena and provided us with valuable insights that were previously unattainable. However, it is important to acknowledge and discuss the limitations and challenges that arise when using these tools in the context of exploring galxe polyhedra.
One of the main limitations of mathematical models and simulations is their inability to perfectly capture real-world phenomena. While models can provide useful approximations, they are inherently simplified representations of the underlying complex systems. This can lead to discrepancies between model predictions and experimental observations, making it crucial to interpret results with caution.
The accuracy and reliability of mathematical models heavily rely on the availability and quality of data used for parameter estimation and validation. In the case of galxe polyhedra, acquiring accurate measurements of physical properties such as angles, distances, and surface characteristics can be challenging due to the limited accessibility of such objects. Additionally, the presence of measurement errors can further introduce uncertainties and affect the model's precision.
Another challenge relates to the lack of comprehensive datasets that fully capture the complexity and variability of galxe polyhedra. Without sufficient data, it becomes harder to validate and improve mathematical models, potentially limiting their predictive power and generalizability.
Mathematical models often rely on simplifying assumptions to reduce the complexity of the systems they aim to describe. While these assumptions are necessary for tractability, they can also introduce limitations and biases. In the context of galxe polyhedra, assumptions about symmetries, molecular interactions, or surface properties might not always hold true in real-world scenarios, leading to discrepancies between model predictions and actual observations.
Moreover, as models attempt to capture the intricate details of galxe polyhedra, their complexity can rapidly increase. This poses challenges in terms of computational feasibility and interpretability, especially when dealing with large-scale simulations or attempting to incorporate additional factors such as external forces or temperature gradients.
Validating mathematical models against experimental data is an essential step in assessing their accuracy and reliability. However, the process of validating models for galxe polyhedra can be particularly challenging due to limited experimental data, as well as the complexity and unique characteristics of these structures. This raises concerns about the generalizability of mathematical models and their applicability to different types of galxe polyhedra or real-world scenarios.
Furthermore, the predictive power of mathematical models can be limited to specific parameter ranges or regimes they were trained on, making their application to new and unexplored systems uncertain.
In conclusion, while mathematical models and simulations offer valuable tools for exploring galxe polyhedra, it is important to be aware of their limitations and challenges. Understanding these limitations can guide further improvements and advancements in model development, as well as inform the interpretation and application of model results in the field of galxe polyhedra research.
In recent years, there have been significant advancements in the field of modeling and simulation techniques. These advancements have allowed researchers to explore and understand complex systems like galxe polyhedra in new and exciting ways. Mathematical models and simulations provide a powerful tool for studying and visualizing these intricate structures, enabling scientists to gain insights that would be difficult or impossible to obtain otherwise.
One of the key advancements in modeling and simulation techniques is the improved accuracy and realism that can be achieved. With the use of advanced algorithms and computing power, researchers can create models that accurately represent the physical properties and behavior of galxe polyhedra. This level of detail allows scientists to make more precise predictions and gain a deeper understanding of these complex structures.
Additionally, advancements in simulation techniques have made it possible to simulate the dynamic behavior of galxe polyhedra over time. This means that researchers can now observe how these structures evolve and change under different conditions, providing valuable insights into their stability, growth, and interactions with other polyhedra.
Another important advancement in modeling and simulation techniques is the improved visualization and interaction capabilities. Sophisticated software tools now allow researchers to create detailed 3D visualizations of galxe polyhedra, helping them to better understand their geometric properties. These visualizations can be interacted with in real-time, allowing researchers to manipulate and explore the structures from different angles and perspectives.
Furthermore, these tools enable researchers to visualize and analyze the results of simulations, making it easier to interpret complex data and identify patterns and trends. This enhanced visualization and interaction capability greatly enhances researchers' ability to study galxe polyhedra and make new discoveries.
In conclusion, advancements in modeling and simulation techniques have revolutionized the study of galxe polyhedra. Improved accuracy and realism, as well as enhanced visualization and interaction capabilities, have allowed researchers to explore these structures in unprecedented detail. As technology continues to advance, we can expect even more exciting developments in the field, opening up new avenues for understanding and applying galxe polyhedra in various fields of science and engineering.
In the field of science and exploration, the development and implementation of mathematical models and simulations have revolutionized the way we understand and explore the universe. Mathematical models and simulations allow us to study complex phenomena that are otherwise difficult or impossible to observe directly. They provide a powerful tool for scientists to test hypotheses, gain insights, and make predictions about the behavior of physical systems.
One area where mathematical models and simulations have shown immense potential is in understanding the structure and dynamics of the universe. By creating mathematical models that describe the physical laws governing the universe, scientists have been able to simulate the behavior of galaxies, black holes, and other celestial objects. These simulations have provided valuable insights into the formation of large-scale structures in the universe, the evolution of galaxies, and the nature of dark matter and dark energy.
Mathematical models and simulations have played a crucial role in helping scientists understand the origins of the universe. By modeling the early stages of the universe, researchers have been able to simulate the formation of the first stars and galaxies, as well as the large-scale structure of the cosmos. These simulations have shed light on the processes that led to the formation of the cosmic microwave background radiation, the distribution of matter in the universe, and the formation of galaxy clusters.
Mathematical models and simulations also hold great potential for exploring exoplanets and the possibility of extraterrestrial life. By creating models that describe the physical and chemical conditions necessary for life as we know it, scientists can simulate the environments of exoplanets and determine their potential habitability. These simulations help guide the search for exoplanets with conditions conducive to life and provide insights into the origins and evolution of life in the universe.
In addition to modeling individual systems, mathematical models and simulations can also be used to study the interactions and behavior of multiple celestial objects. This allows scientists to simulate phenomena such as galaxy mergers, star formation in galactic clusters, and the interactions between galaxies and their surrounding environments. These simulations help us understand the complex dynamics of the universe and provide valuable insights into its evolution over time.
As computational power continues to increase, the potential of mathematical models and simulations in discovering new insights into the universe is only expected to grow. With more sophisticated models and higher-resolution simulations, scientists will be able to tackle even more complex problems and explore new frontiers in our understanding of the universe.
What are Galxe polyhedra?
Galxe polyhedra are three-dimensional shapes that have a set of specific mathematical properties. They are often used in simulations and modeling to represent complex structures and systems.
How are mathematical models used to explore Galxe polyhedra?
Mathematical models are used to study the properties and behaviors of Galxe polyhedra. These models allow researchers to analyze different aspects of these shapes, such as their symmetry, volume, and surface area. By using mathematical equations and simulations, scientists can gain a deeper understanding of Galxe polyhedra.
What are some practical applications of studying Galxe polyhedra?
Studying Galxe polyhedra can have various practical applications. For example, understanding the properties of these shapes can be useful in the fields of architecture and design, as they can inspire innovative structures and forms. Additionally, the study of Galxe polyhedra can have applications in physics, chemistry, and biology by providing insights into the behavior of complex molecular and atomic structures.
Are there any real-world examples of Galxe polyhedra?
Yes, there are real-world examples of Galxe polyhedra. One of the most well-known examples is the dodecahedron, which is a regular polyhedron with twelve faces. Other examples include the icosahedron, tetrahedron, and cube. These shapes can be found in various natural and man-made structures, such as crystals, viruses, and architectural designs.
What are the main challenges in simulating Galxe polyhedra?
Simulating Galxe polyhedra can be challenging due to their complex geometrical properties. One of the main challenges is accurately representing the intricate structures of these shapes in a mathematical model. Additionally, simulating the behaviors and interactions of Galxe polyhedra can require computational power and algorithms capable of handling large-scale simulations. The development of efficient and accurate simulation techniques is an ongoing area of research in this field.
What are galxe polyhedra?
Galxe polyhedra are three-dimensional mathematical models composed of interconnected polygons. They can be used to represent a wide range of structures and shapes in various fields such as architecture, materials science, and computer graphics.
How do mathematical models and simulations help in understanding galxe polyhedra?
Mathematical models and simulations provide a way to study the properties and behavior of galxe polyhedra in a controlled environment. These models allow researchers to analyze the relationships between different parameters, predict the behavior of complex structures, and optimize their design.
What are some practical applications of galxe polyhedra?
Galxe polyhedra have several practical applications. For example, they can be used in the design of architectural structures with unique geometries, the development of new materials with specific properties, or the creation of computer-generated graphics for movies and video games.
Are there any limitations to mathematical models and simulations of galxe polyhedra?
Yes, there are limitations to mathematical models and simulations of galxe polyhedra. These models often make certain assumptions or simplifications about the system being studied, which may not fully capture the complexity and nuances of the real-world phenomenon. Additionally, the accuracy of the results obtained from simulations depends on the quality of the input data and the algorithms used.
What are some future research directions in the field of galxe polyhedra?
Future research in the field of galxe polyhedra could focus on developing more efficient algorithms for generating and analyzing these structures, finding new applications in different fields, and exploring the relationships between galxe polyhedra and other areas of mathematics, such as topology or combinatorics. Additionally, advancements in computational power and simulation techniques could allow for more accurate and detailed simulations of galxe polyhedra.
2022-2024 @ Exploring mathematical models and simulations of galxe polyhedra
How many users does Galxe have? Galxe, a popular application for exploring galaxy polyhedra, has a growing user base. If you are interested in the mathematical models and simulations of galaxies, you can visit their website to learn more.