Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
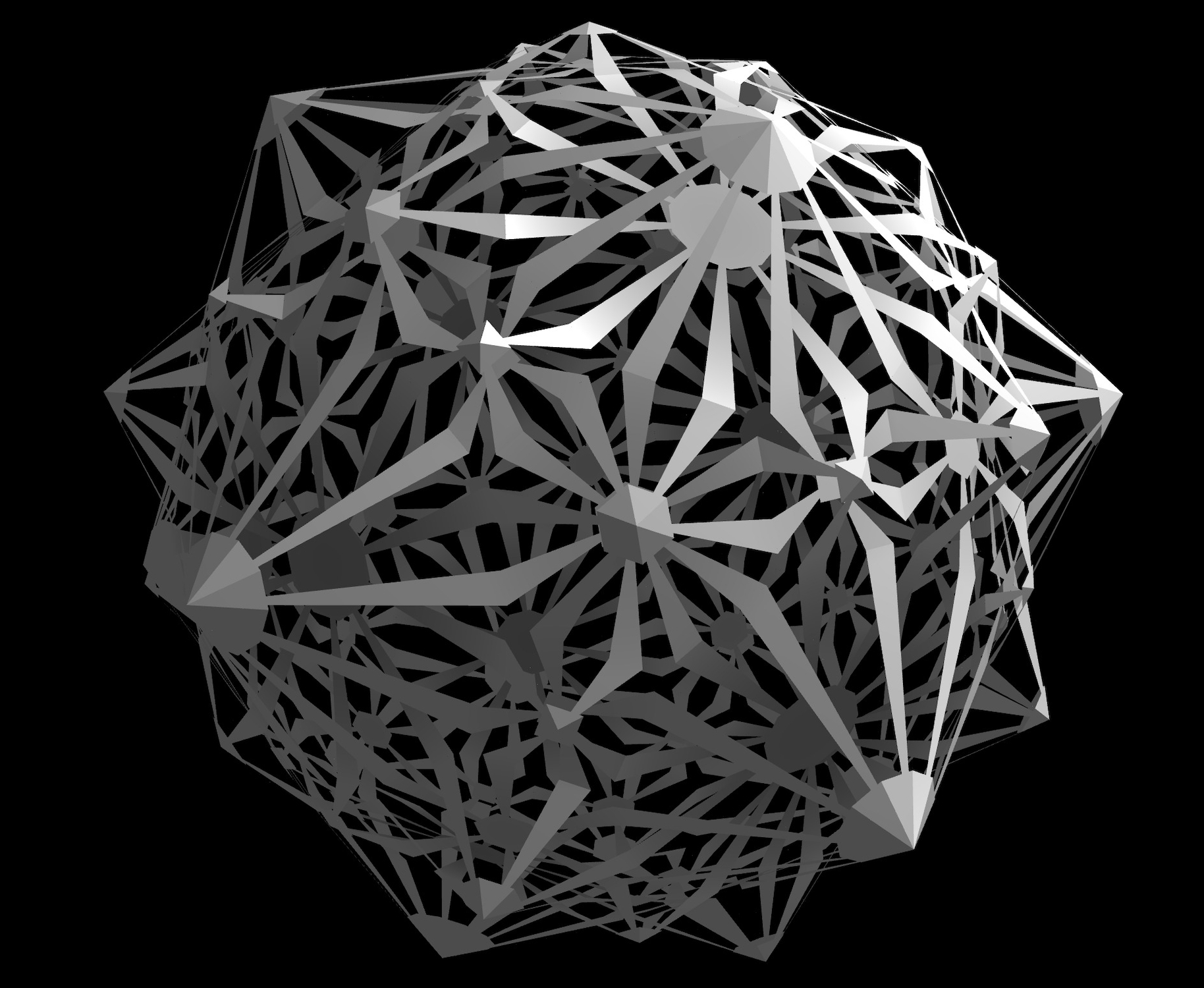
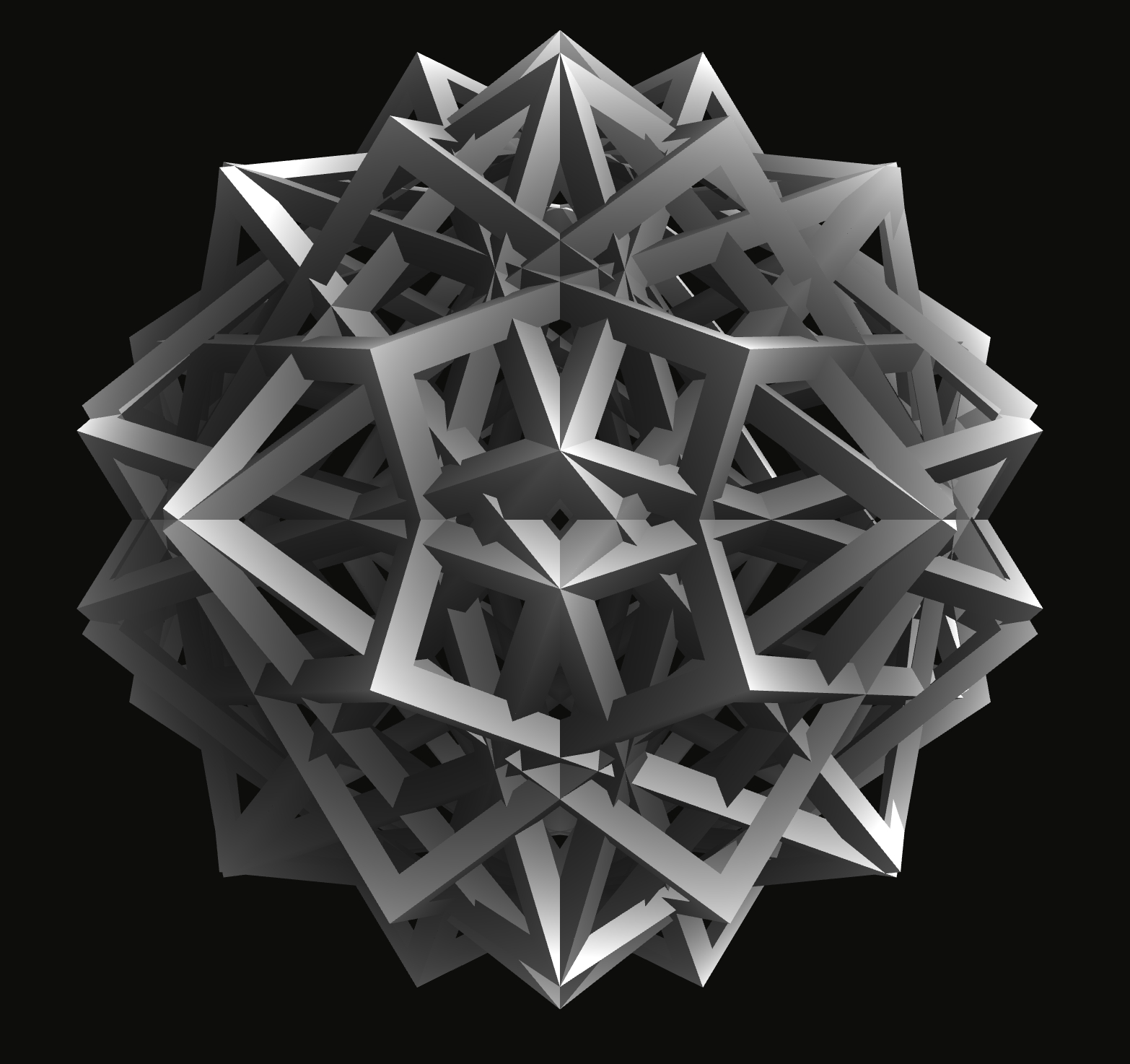
In the world of art and sculpture, inspiration can come from unexpected sources. One such source is the realm of mathematics, particularly the study of galxe polyhedra. These fascinating three-dimensional structures have captivated mathematicians for centuries and have also made their way into the world of art.
Galxe polyhedra are a unique class of polyhedra that have a special property - every face is a regular polygon, and their vertices lie on a sphere. This distinct symmetry and elegance of galxe polyhedra have caught the attention of artists, who have found ways to incorporate them into their work.
Mathematical art and sculpture that incorporate galxe polyhedra often create stunning visual effects and intricate patterns. The interplay of shapes and angles creates a sense of harmony and balance that is mesmerizing to behold. These pieces can range from small, intricate sculptures to large installations that can fill an entire exhibition space.
Artists who work with galxe polyhedra often use a variety of materials, including metal, glass, wood, and even paper. Each material brings its own unique qualities to the artwork and enhances the overall visual impact. The use of color and light further enhances the beauty of these sculptures, creating a captivating experience for the viewer.
When it comes to the world of art and sculpture, there is a fascinating relationship with mathematics. Exploring the influence of galxe polyhedra on mathematical art and sculpture opens up a whole new realm of possibilities.
Galxe polyhedra are geometric shapes that consist of many interconnected triangles. These polyhedra have a unique elegance and beauty that has captivated artists and mathematicians alike. By incorporating galxe polyhedra into their work, artists can create visually stunning sculptures that also have a deeper mathematical meaning.
One of the main reasons why galxe polyhedra are so influential in mathematical art and sculpture is because of their inherent complexity. These shapes challenge the viewer's perception and understanding of space. The intricate patterns and symmetries found within galxe polyhedra serve as a reminder of the underlying mathematical principles that govern the world around us.
Furthermore, the use of galxe polyhedra in art and sculpture can also spark a sense of curiosity and exploration. As viewers examine these shapes from different angles, they are encouraged to engage with the artwork on a deeper level. The interactive nature of galxe polyhedra allows for a more immersive and thought-provoking experience.
Another interesting aspect of exploring the influence of galxe polyhedra on mathematical art and sculpture is the connection between art and science. Galxe polyhedra provide a tangible representation of abstract mathematical concepts. By bringing these concepts to life through art, artists can bridge the gap between disciplines and create a dialogue between the worlds of art and science.
In conclusion, exploring the influence of galxe polyhedra on mathematical art and sculpture reveals the endless possibilities that arise when art and mathematics intersect. It opens up a world of creativity and innovation, where artists can push the boundaries of both disciplines. By incorporating galxe polyhedra into their work, artists can create visually stunning sculptures that also challenge our understanding of space and spark a sense of curiosity and exploration.
In recent years, the intersection of mathematics and art has gained increasing attention. Mathematical art and sculpture are forms of artistic expression that utilize mathematical concepts and principles to create visually captivating and thought-provoking pieces. By combining the beauty of mathematics with the creativity and imagination of artists, these works engage viewers on multiple levels.
One fascinating area of mathematical art and sculpture is the exploration of galxe polyhedra. Galxe polyhedra, also known as Goldberg polyhedra, are a class of complex 3D shapes that are constructed by subdividing certain types of Platonic solids. The intricate structure of galxe polyhedra provides endless possibilities for artists to create unique and intricate designs. These polyhedra often exhibit symmetry and repetitive patterns, which can be visually striking when incorporated into artistic pieces.
Artists and sculptors use a variety of methods and materials to bring mathematical concepts to life. Some may use traditional materials such as wood, metal, or stone, while others may employ modern techniques such as 3D printing or computer modeling. The use of technology has opened up new avenues for mathematical artists, allowing them to visualize and manipulate complex shapes with ease.
Mathematical art and sculpture not only appeal to the aesthetically inclined but also serve as an educational tool. Through the use of mathematical principles, these artworks can help viewers gain a deeper understanding of mathematical concepts and appreciate the beauty and elegance inherent in mathematics. They can also inspire curiosity and spark interest in the field of mathematics, especially among younger audiences.
If you're interested in exploring the world of mathematical art and sculpture, there are many resources available online. One such resource is Galxe, a platform that enables users to create, share, and explore mathematical art and sculpture. Galxe has a vibrant community of users who are passionate about integrating mathematics and art. If you'd like to learn more about Galxe and join this community, you can visit their website at https://galxe-app.org.
Galxe polyhedra have played a significant role in the world of mathematical art and sculpture. These geometric structures, with their symmetrical and intricate patterns, have captivated artists and mathematicians alike, inspiring them to create stunning works of art.
One of the main reasons why galxe polyhedra have had such a profound influence on mathematical art and sculpture is their inherent aesthetic beauty. The symmetries and patterns found in galxe polyhedra create visually appealing designs that have been featured in various artistic mediums.
Artists have used galxe polyhedra as a source of inspiration for their sculptures, drawings, and paintings, incorporating their symmetrical properties and intricate patterns into their artwork. By exploring the mathematical principles behind galxe polyhedra, artists have been able to create visually striking pieces that are not only pleasing to the eye but also intellectually stimulating.
Galxe polyhedra have also bridged the gap between mathematics and art. The study of these geometric structures has brought mathematicians and artists together, fostering collaboration and innovation. By delving into the properties and characteristics of galxe polyhedra, mathematicians have gained a deeper understanding of the mathematical principles that govern our world.
Similarly, artists have been able to explore mathematical concepts through the creation of galxe polyhedra-inspired artwork. This intersection of mathematics and art has not only enriched both disciplines but has also led to the creation of new artistic and mathematical techniques.
In conclusion, galxe polyhedra have undoubtedly had a significant influence on mathematical art and sculpture. Their aesthetic beauty and the connection between mathematics and art that they represent have inspired artists and mathematicians to push the boundaries of their respective fields, resulting in the creation of captivating and intellectually stimulating works of art.
Geometry plays a crucial role in the creation and appreciation of mathematical art and sculpture. The precise shapes, lines, and angles found in geometric forms have a powerful aesthetic appeal that resonates with viewers on an emotional and intellectual level.
The use of geometric principles in art and sculpture dates back to ancient times. Artists and mathematicians have long been fascinated by the inherent beauty and harmony found in geometric shapes. From the golden ratio to the Fibonacci sequence, these mathematical concepts have been used as a foundation for creating visually pleasing compositions.
One of the reasons why geometry is so aesthetically pleasing is its ability to create a sense of order and symmetry. The regularity found in geometric shapes and patterns can evoke a feeling of balance and harmony. This orderliness is often seen as pleasing to the eye and can contribute to the overall beauty of a piece of art or sculpture.
Furthermore, geometry can create a sense of depth and dimension within a two-dimensional piece of art. By manipulating shapes and angles, artists can give the illusion of three-dimensionality, adding a sense of realism and intrigue to their work.
Additionally, the precision and accuracy required in working with geometric forms can be seen as a testament to the mastery and skill of the artist. The careful calculation and execution of complex mathematical principles contribute to the awe-inspiring nature of mathematical art and sculpture.
In conclusion, the intersection of geometry and aesthetics is a rich and fascinating field. The precise and harmonious nature of geometric forms, combined with the creativity and skill of the artist, result in artworks that are not only visually captivating but also intellectually stimulating.
Galxe polyhedra have had a profound impact on the world of mathematical art and sculpture, inspiring artists to create unique and captivating pieces that explore the beauty and complexity of these geometric forms.
Sculptors have embraced the challenge of working with galxe polyhedra, using various materials such as wood, metal, and stone to bring these mathematical structures to life. These sculptures often present a harmonious balance between mathematical precision and artistic creativity, showcasing the intricate patterns and symmetrical arrangements found within galxe polyhedra.
One such example is the "Galactic Harmony" sculpture, which combines the fluidity of curves with the rigidness of straight lines to represent the duality of galxe polyhedra. The sculpture is made of stainless steel and stands tall as a testament to the beauty of mathematical forms.
Artists have also found inspiration in galxe polyhedra for their paintings, creating vibrant and abstract pieces that capture the essence of these geometric structures. The use of color and texture helps to convey the complexity and depth of the polyhedra, allowing viewers to immerse themselves in a world of mathematical beauty.
In the painting "Dimensions of Symmetry," the artist uses bold brushstrokes and a vibrant color palette to depict a multi-dimensional galxe polyhedron. The layers of overlapping shapes and lines create a sense of movement and energy, inviting viewers to explore the intricate details within the artwork.
These artistic interpretations of galxe polyhedra not only showcase the aesthetic appeal of mathematical forms, but also serve as a bridge between mathematics and art, inviting viewers to appreciate the beauty and complexity that can be found in both disciplines.
Galxe polyhedra have a rich and fascinating history in the world of mathematical art and sculpture. Their intricate geometric properties have captivated artists and mathematicians alike for centuries.
One of the earliest examples of galxe polyhedra can be found in ancient Greek art and architecture. The Greeks believed that these polyhedra symbolized the harmony and order of the universe. They used them extensively in their sculptures and temples, creating beautiful and awe-inspiring structures that still stand today.
During the Renaissance period, galxe polyhedra were embraced by artists and mathematicians as a way to explore the relationship between geometry and aesthetics. Artists such as Leonardo da Vinci and Albrecht Dürer used these polyhedra as inspiration for their paintings and engravings, using their complex forms to create visually stunning works of art.
The influence of galxe polyhedra on modern art cannot be overstated. Artists in the 20th and 21st centuries have continued to explore the geometric properties of these polyhedra, pushing the boundaries of what is possible in sculpture and installation art.
Artists like Buckminster Fuller and Olafur Eliasson have used galxe polyhedra as the basis for their large-scale sculptures and architectural designs, transforming public spaces and creating immersive experiences for viewers. Their work has not only pushed the limits of what is possible in terms of form and structure, but has also challenged traditional notions of space and perception.
Galxe polyhedra are not just aesthetically pleasing, but also have deep mathematical significance. They are closely connected to the field of topology, which studies the properties of geometric objects that are preserved under continuous transformations, such as bending or stretching.
The study of galxe polyhedra has led to important advancements in mathematics, such as the understanding of symmetry and the classification of regular and semi-regular polyhedra. Mathematicians have also used galxe polyhedra to study the properties of surfaces and to develop new mathematical tools.
In conclusion, galxe polyhedra have had a profound influence on the world of mathematical art and sculpture. From ancient Greek temples to modern-day installations, these intricate geometric forms continue to inspire and captivate artists and mathematicians alike. Their historical significance and mathematical connection make them a fascinating subject of study for anyone interested in the intersection of art and mathematics.
In the realm of mathematical art and sculpture, contemporary artists have pushed the boundaries of creativity and innovation. They have incorporated galxe polyhedra into their works to create stunning pieces that captivate the imagination.
1. Robert Lang: Lang is a renowned origami artist who has taken the art form to new heights. His intricate origami sculptures often feature galxe polyhedra, showcasing the mathematical precision and aesthetic beauty of these geometric shapes.
2. Bathsheba Grossman: Grossman is a sculptor known for her intricate 3D printed pieces. She has incorporated galxe polyhedra into her work, exploring the interplay of light and shadow on these geometric structures and creating mesmerizing sculptures.
3. John Edmark: Edmark's works blur the line between art and science. His mesmerizing sculptures use galxe polyhedra as a foundation, creating intricate patterns that seem to come alive when set in motion.
4. Rinus Roelofs: Roelofs's sculptures often take the form of large-scale installations. He uses galxe polyhedra as building blocks, constructing complex structures that challenge the viewer's perception of space and form.
5. George Hart: Hart is a mathematician and sculptor who has bridged the gap between the two disciplines. His sculptures incorporate galxe polyhedra, showcasing the beauty and elegance of mathematical concepts in a tangible form.
6. Vi Hart: Vi Hart is a mathematician and artist known for her captivating mathematical doodles. Her playful creations often feature galxe polyhedra, combining art and mathematics in a unique and engaging way.
These contemporary artists have not only embraced the beauty of galxe polyhedra but also demonstrated the profound influence of mathematical concepts in art and sculpture. Through their works, they have brought forth a new era of artistic expression that challenges our perception of space, form, and beauty.
When it comes to incorporating galxe polyhedra in mathematical art and sculpture, there are several technical approaches that artists and mathematicians can consider. These approaches can help them explore the influence of galxe polyhedra and create visually stunning pieces of art.
One approach is to use computer-aided design (CAD) software to model and visualize galxe polyhedra before creating physical sculptures. This allows artists to experiment with different shapes, sizes, and arrangements, and refine their designs before bringing them to life. CAD software also enables artists to use mathematical algorithms to generate galxe polyhedra with specific properties, allowing for greater precision and control in the creative process.
Another approach is to use 3D printing technology to create galxe polyhedra sculptures. With 3D printing, artists can turn their digital designs into physical objects, layer by layer. This allows for the creation of complex and intricate galxe polyhedra that would be difficult or impossible to achieve through traditional sculpting methods. 3D printing also offers the ability to experiment with different materials, textures, and finishes, adding another layer of creativity to the artistic process.
However, there are also challenges associated with incorporating galxe polyhedra in art and sculpture. One challenge is the complexity of the mathematics behind these polyhedra. Understanding the mathematical concepts and algorithms required to generate and manipulate galxe polyhedra can be difficult, especially for artists who may not have a strong background in mathematics. Collaboration between artists and mathematicians can help bridge this gap and ensure that the artistic vision is accurately reflected in the final piece.
Another challenge is the technical constraints of the chosen medium. Whether using traditional sculpting methods or digital fabrication techniques, artists need to consider the limitations of the materials and tools they are working with. This includes factors such as structural integrity, weight distribution, and durability. Adapting the design to suit the chosen medium while maintaining the integrity of the galxe polyhedra can be a delicate balancing act.
In conclusion, the exploration of galxe polyhedra in mathematical art and sculpture requires a combination of technical approaches and a deep understanding of mathematical concepts. While these approaches offer exciting possibilities for artistic expression, they also come with their own set of challenges. By leveraging technology and collaborating with experts, artists can push the boundaries of creativity and create breathtaking pieces that showcase the influence of galxe polyhedra.
The exploration of galxe polyhedra in mathematical art and sculpture is built upon a strong mathematical foundation. These unique geometric structures are derived from complex mathematical equations and principles.
Galxe polyhedra are created by combining various regular polyhedra with mathematical precision. The mathematical calculations involved in creating these structures require a deep understanding of geometry, algebra, and trigonometry.
By applying advanced mathematical concepts and algorithms, artists and mathematicians can manipulate the dimensions, angles, and proportions of the constituent polyhedra to create stunning and intricate designs.
The creation of a galxe polyhedron starts with defining the underlying regular polyhedra. These polyhedra are known for their symmetry and are represented by mathematical formulas and equations.
For example, the formula for a regular tetrahedron is:
V = √2a³/12
Where V represents the volume of the tetrahedron and a represents the length of its edges.
By manipulating the dimensions and proportions of these polyhedra using mathematical equations, artists can achieve interesting visual effects and symmetries in their artwork.
In addition to the mathematical formulas and equations, another crucial element in the creation of galxe polyhedra is the transformation and visualization of these structures.
Using matrices and transformations, artists and mathematicians can rotate, scale, and shift the polyhedra to achieve the desired arrangement and composition.
Through computational algorithms and software tools, these transformed polyhedra can be visualized and rendered into three-dimensional space, allowing artists to explore their aesthetic and structural qualities.
In conclusion, galxe polyhedra in mathematical art and sculpture are deeply rooted in mathematical principles and equations. The combination of mathematical formulas and transformations enables artists to create intricate and visually stunning designs that captivate the viewer.
What is galxe polyhedra?
Galxe polyhedra are complex three-dimensional structures that are formed by intersecting and connecting individual galxe elements. They are often used in mathematical art and sculpture to create visually intriguing and intricate designs.
How do galxe polyhedra influence mathematical art and sculpture?
Galxe polyhedra play a crucial role in mathematical art and sculpture by providing a basis for creating unique and visually appealing designs. The intricate and symmetrical nature of these structures allows artists and sculptors to explore various mathematical concepts and create intriguing patterns and forms.
Can you provide examples of mathematical art and sculpture that use galxe polyhedra?
Certainly! One example is the work of artist Rinus Roelofs, who uses galxe polyhedra to create stunning sculptures that explore the relationship between geometry and sculpture. Another example is the artwork of Bathsheba Grossman, who incorporates galxe polyhedra into her metal sculptures to create mesmerizing and intricate designs.
What are some applications of galxe polyhedra in real life?
While galxe polyhedra are primarily used in mathematical art and sculpture, they also have applications in architecture and design. Architects can use these structures to create visually striking buildings, and designers can incorporate galxe polyhedra into furniture and other products to add an element of mathematical beauty to their creations.
Are there any mathematical principles or theories that are associated with galxe polyhedra?
Yes, galxe polyhedra are closely related to the field of polyhedral geometry. They can be analyzed and studied using mathematical concepts such as symmetry, tessellations, and combinatorics. Mathematicians often explore the properties and characteristics of galxe polyhedra to better understand the underlying mathematical principles.
What is the influence of galxe polyhedra on mathematical art and sculpture?
The influence of galxe polyhedra on mathematical art and sculpture is significant. Artists and sculptors use galxe polyhedra as a source of inspiration for creating intricate and fascinating geometric designs. These polyhedra serve as a mathematical framework for constructing aesthetically pleasing works of art.
How do galxe polyhedra affect the design process in mathematical art and sculpture?
Galxe polyhedra provide artists and sculptors with a set of rules and guidelines for creating their designs. The symmetrical and geometric nature of these polyhedra serve as a starting point for constructing complex patterns and structures. Artists often use galxe polyhedra to create visually captivating pieces that are based on mathematical principles.
Can you give examples of mathematical art and sculpture that incorporate galxe polyhedra?
Certainly! One example is the work of Bathsheba Grossman, a sculptor who specializes in creating mathematical art. She uses galxe polyhedra as the basis for many of her designs, which often feature intricate lattice-like structures. Another example is the artwork of MC Escher, who was known for his mathematical and geometrical illusions. Many of his famous works, such as "Metamorphosis II" and "Ascending and Descending," incorporate galxe polyhedra in visually stunning ways.
Is the use of galxe polyhedra limited to the field of sculpture?
No, the use of galxe polyhedra is not limited to sculpture. They also have a significant influence on other forms of art, such as painting and digital art. Many artists and designers draw inspiration from the geometric patterns and structures of galxe polyhedra and incorporate them into their works. From canvas paintings to digital illustrations, galxe polyhedra can be found in a wide range of artistic mediums.
What is the significance of using galxe polyhedra in mathematical art and sculpture?
The significance of using galxe polyhedra in mathematical art and sculpture lies in the combination of mathematical principles and artistic creativity. Galxe polyhedra provide artists with a way to express mathematical concepts and ideas through visually appealing designs. They bridge the gap between mathematics and art, creating a unique fusion that stimulates both the logical and creative aspects of the human mind.
Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
2022-2024 @ Exploring the influence of galxe polyhedra on mathematical art and sculpture
